Understanding how to calculate the circumference of a circle is a fundamental aspect of geometry that has applications in various fields, from engineering and architecture to everyday situations. Whether you’re a student grappling with geometry homework, a professional needing precision in your projects, or just someone curious about the mathematical fabric of the world around us, this guide will walk you through the concept, formula, and practical applications of finding a circle’s circumference.
Understanding Circumference
Before diving into calculations, let’s clarify what we mean by the circumference of a circle. The circumference is the distance around a circle’s edge or perimeter. It’s the circular equivalent of the perimeter of polygons, providing a measure of the total distance one would cover if they walked around the circle once. This concept is not just a mathematical abstraction but a practical tool used in various applications, from designing wheels and gears to mapping out circular tracks and fields.
The Formula for Circumference
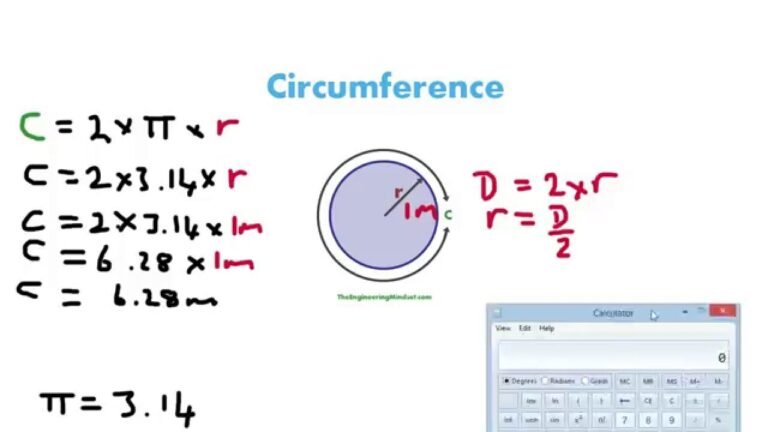
The circumference of a circle can be found using a simple formula that involves the circle’s diameter or radius and the mathematical constant π (pi). Pi is a crucial part of this calculation, an irrational number approximately equal to 3.14159, representing the ratio of any circle’s circumference to its diameter. The formulae to find the circumference are as follows:
- Using the Diameter: The circumference (C) is equal to π times the diameter (D) of the circle.
- �=��
- C=πD
- Using the Radius: Since the diameter is twice the radius (R), the formula can also be expressed as the circumference being 2 times π times the radius.
- �=2��
- C=2πR
These formulas are the cornerstone of calculating the circumference, allowing for straightforward computation as long as you know the circle’s diameter or radius.
Step-by-Step Calculation
To make this more concrete, let’s go through the steps of calculating the circumference using both the diameter and the radius.
Using the Diameter
- Measure the Diameter: Use a ruler or a measuring tape to find the distance across the circle through its center point. This measurement is the diameter.
- Apply the Formula: Multiply the diameter by π (3.14159). For instance, if the diameter is 10 units, the circumference is
- 10×�≈31.4159
- 10×π≈31.4159 units.
Using the Radius
- Measure the Radius: Measure the distance from the center of the circle to any point on its edge. This measurement is the radius.
- Apply the Formula: Multiply the radius by 2 and then by π. If the radius is 5 units, the circumference is
- 2×5×�≈31.4159
- 2×5×π≈31.4159 units.
Practical Applications
The knowledge of how to find the circumference of a circle has practical applications in numerous fields. In engineering, it’s essential for designing components that fit together perfectly in machinery or for creating circular objects like pipes, wheels, and gears. Architects use this calculation when designing circular structures, ensuring the materials align correctly. It’s also vital in sports, for instance, in determining the distance around circular tracks or fields. Everyday examples include finding the right size of round tablecloths, calculating the length of fencing needed for a circular garden, or even wrapping a gift with a circular base efficiently.
Troubleshooting Common Problems
While the process is straightforward, errors can occur, usually due to incorrect measurement or calculation. Always double-check your measurements and ensure you’re using the correct formula. Remember that precision in measurement is key, especially in professional applications where even slight inaccuracies can lead to significant issues.
Conclusion
Finding the circumference of a circle is a simple yet powerful mathematical skill that has wide-ranging applications. By understanding and applying the correct formula, you can unlock the potential of circular measurements in both academic and real-world scenarios. Whether you’re calculating for homework, professional projects, or just out of curiosity, the ability to determine a circle’s circumference is an invaluable tool in your mathematical toolkit.